Cycle detection in Graph: C Program implementation
6. Cycle Detection in Graphs Challenges
Write a C program that implements a function in C to check whether a given graph contains a cycle or not.
From Wikipedia,
In graph theory, a cycle in a graph is a non-empty trail in which only the first and last vertices are equal. A directed cycle in a directed graph is a non-empty directed trail in which only the first and last vertices are equal.
A graph without cycles is called an acyclic graph. A directed graph without directed cycles is called a directed acyclic graph. A connected graph without cycles is called a tree.
Here is a graph with edges colored to illustrate a closed walk, H–A–B–A–H, in green; a circuit which is a closed walk in which all edges are distinct, B–D–E–F–D–C–B, in blue; and a cycle which is a closed walk in which all vertices are distinct, H–D–G–H, in red.
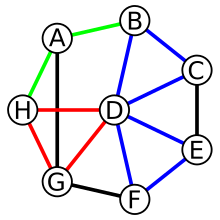
Source: Wikipedia
Sample Solution:
C Code:
#include <stdio.h>
#include <stdlib.h>
#define MAX_VERTICES 100
// Function to add an edge to the graph
void addEdge(int graph[MAX_VERTICES][MAX_VERTICES], int start, int end) {
graph[start][end] = 1;
graph[end][start] = 1; // For undirected graph
}
// Recursive function to perform DFS and check for cycles
int isCyclicUtil(int graph[MAX_VERTICES][MAX_VERTICES], int currentVertex, int parent, int visited[MAX_VERTICES]) {
visited[currentVertex] = 1;
for (int i = 0; i < MAX_VERTICES; i++) {
if (graph[currentVertex][i]) {
if (!visited[i]) {
if (isCyclicUtil(graph, i, currentVertex, visited))
return 1;
} else if (i != parent) {
return 1;
}
}
}
return 0;
}
// Function to check whether a graph contains a cycle
int isCyclic(int graph[MAX_VERTICES][MAX_VERTICES], int vertices) {
int visited[MAX_VERTICES] = {0};
for (int i = 0; i < vertices; i++) {
if (!visited[i]) {
if (isCyclicUtil(graph, i, -1, visited))
return 1;
}
}
return 0;
}
int main() {
int vertices, edges;
// Input the number of vertices
printf("Input the number of vertices: ");
scanf("%d", &vertices);
if (vertices <= 0 || vertices > MAX_VERTICES) {
printf("Invalid number of vertices. Exiting...\n");
return 1;
}
int graph[MAX_VERTICES][MAX_VERTICES] = {0}; // Initialize the adjacency matrix with zeros
// Input the number of edges
printf("Input the number of edges: ");
scanf("%d", &edges);
if (edges < 0 || edges > vertices * (vertices - 1) / 2) {
printf("Invalid number of edges. Exiting...\n");
return 1;
}
// Input edges and construct the adjacency matrix
for (int i = 0; i < edges; i++) {
int start, end;
printf("Input edge %d (start end): ", i + 1);
scanf("%d %d", &start, &end);
// Validate input vertices
if (start < 0 || start >= vertices || end < 0 || end >= vertices) {
printf("Invalid vertices. Try again.\n");
i--;
continue;
}
addEdge(graph, start, end);
}
// Check if the graph contains a cycle
if (isCyclic(graph, vertices))
printf("The graph contains a cycle.\n");
else
printf("The graph does not contain a cycle.\n");
return 0;
}
Output:
Input the number of vertices: 4 Input the number of edges: 4 Input edge 1 (start end): 0 1 Input edge 2 (start end): 1 2 Input edge 3 (start end): 2 3 Input edge 4 (start end): 3 0 The graph contains a cycle. Note: Here we have a simple cycle with four vertices: 0 → 1 → 2 → 3 → 0.
Explanation:
In the exercise above,
- addEdge Function:
- Adds an undirected edge between two vertices in the graph.
- isCyclicUtil Function:
- Recursive function that performs DFS traversal and checks for cycles.
- It maintains an array 'visited' to mark visited vertices.
- If a vertex i is not visited, it recursively calls the function for i.
- If a vertex i is visited and is not the parent of the current vertex, then there is a cycle.
- isCyclic Function:
- Initiates DFS traversal for each unvisited vertex.
- Calls "isCyclicUtil()" for each unvisited vertex.
- If "isCyclicUtil()" returns true for any vertex, it means a cycle is present in the graph.
- Main Function:
- Takes input for the number of vertices and edges in the graph.
- Constructs the adjacency matrix for the graph by taking input for each edge.
- Calls "isCyclic()" function to check for the presence of a cycle.
- Prints whether the graph contains a cycle or not.
Flowchart:
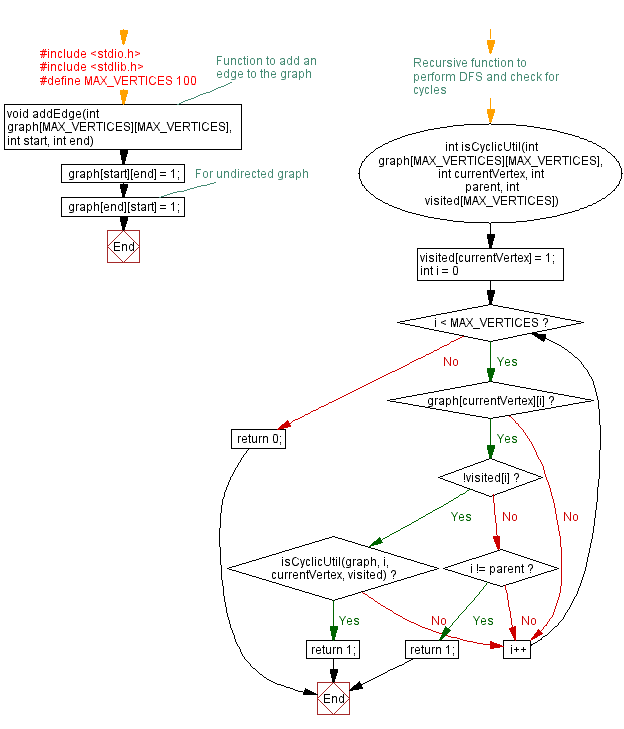
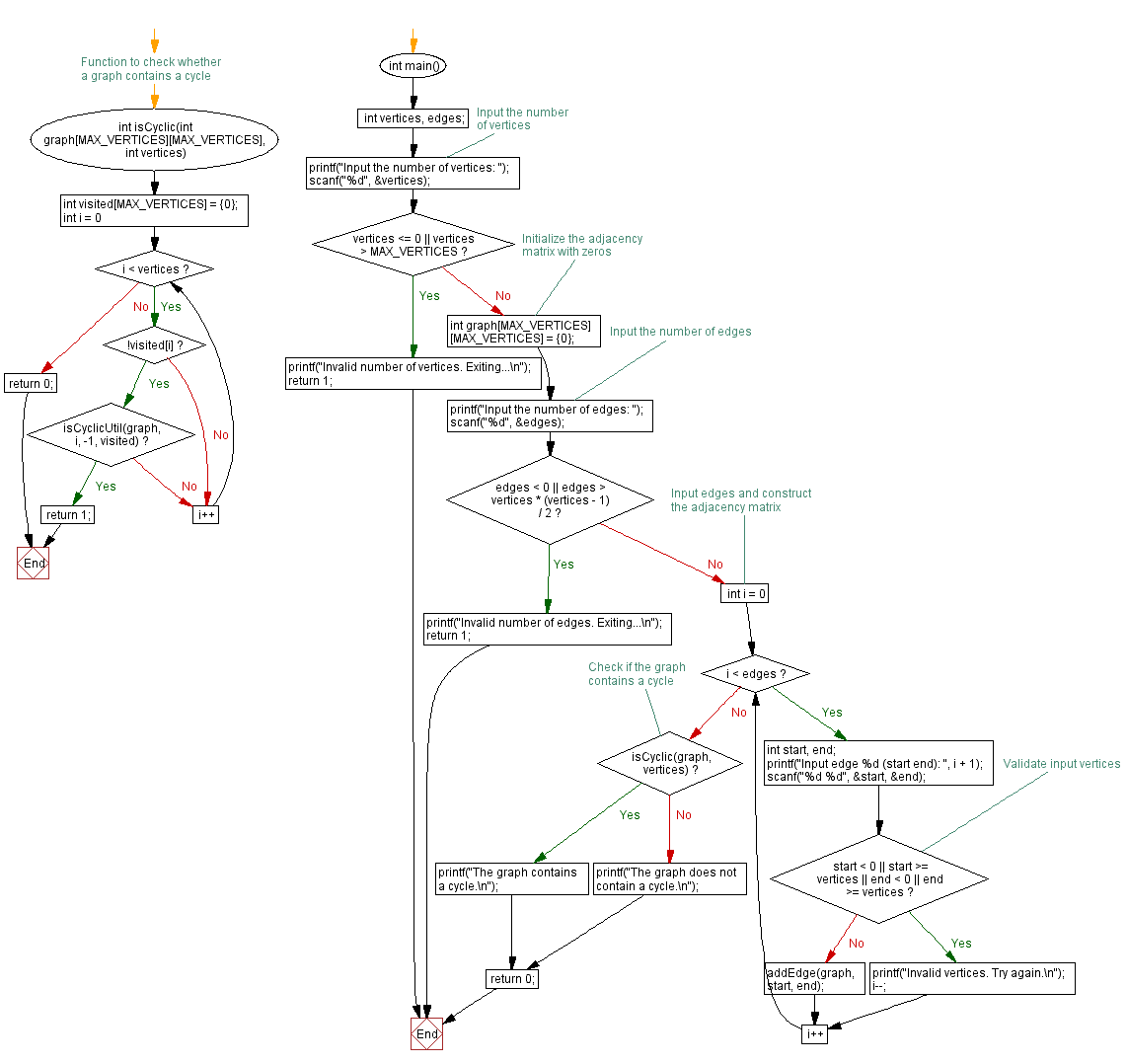
For more Practice: Solve these Related Problems:
- Write a C program to detect cycles in a directed graph using DFS and identify the vertices forming the cycle.
- Write a C program to check for cycles in an undirected graph using the union-find algorithm.
- Write a C program to implement cycle detection using both DFS and BFS methods and compare their outputs.
- Write a C program to detect and print all cycles present in a given graph.
Go to:
PREV : Breadth-First Search (BFS) Extended Challenges.
NEXT : Topological Sorting Extended Challenges.
C Programming Code Editor:
Have another way to solve this solution? Contribute your code (and comments) through Disqus.
What is the difficulty level of this exercise?
Test your Programming skills with w3resource's quiz.
